
Assignment #2: Investigation of the parabola formed by the following equation:

by
Laura Singletary
In order to begin our investigation, let us evaluate a graph of the following equation:
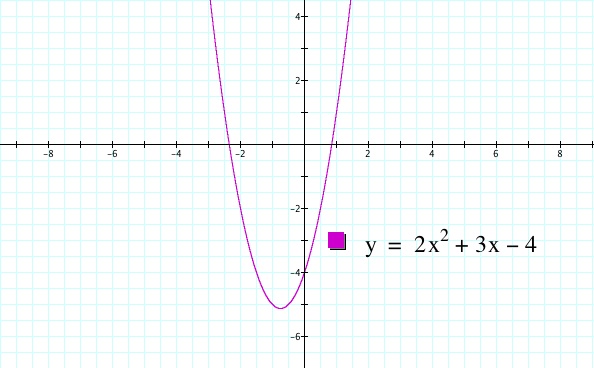
Next, let us overlay a new graph replacing each x by (x - 4). By considering the graphs displayed below, we can evaluate the similarities and the differences that exist between these graphs.
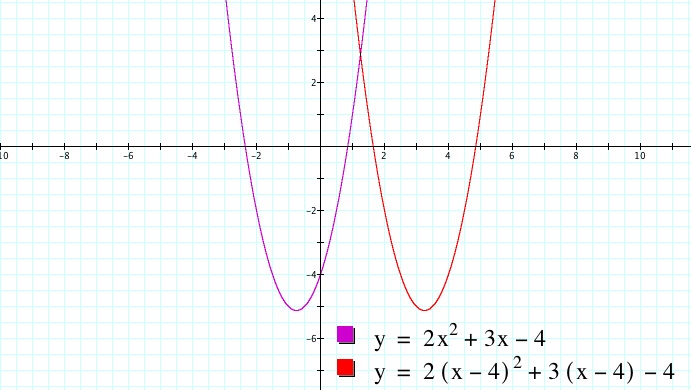
After evaluating both of the graphs, it seems clear that the parabola formed by inserting (x - 4) is shifted four units to the right.
It might seem counterintuitive that inserting (x - 4) would shift the graph to the right. Therefore, let us consider exploring what would happen when we evaluate both equations when x = 2.
The first (purple) equation: y = 2(2)^2 + 3(2) - 4 = 10
The second (red) equation: y = 2(2 - 4)^2 +3(2 - 4) - 4= -2
Obviously, while the x-values were the same, the y-values were different. Why did this happen? When we subtracted 4 from the x-value of 2, our result was the same y-value we would have received if we had evaluated the function at x = -2.
Look at how the graph shifted.
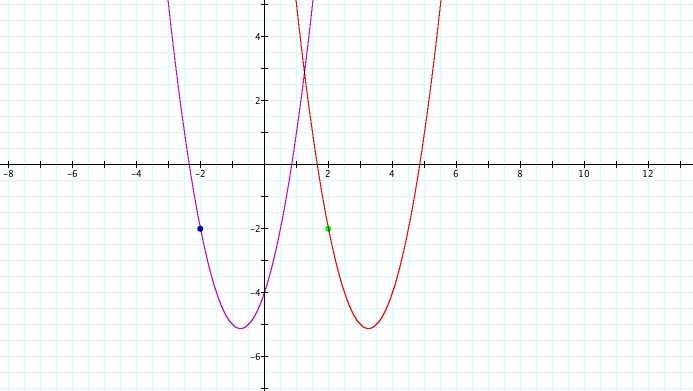
Therefore, by subtracting 4 from the x-value moves the y-values 4 units from the left, which results a translation of the original function 4 units to the right.
Next, let us investigate how we may move the vertex of the original graph into the second quadrant.
Currently, the location of the vertex of our original equation is at the point (-0.75, -5.125). In order to move the vertew of the original equation into the second quadrant, we must shift (or translate) the graph up more than 5.125 units. Let us try shifting the graph upward by 6 units so that the vertex is in the second quadrant. Typically, most people think that in order to accomplish this goal we must add 6 to the y-value. Adding 6 to y is the same as subtracting 6 from the right side of our equation.
Therefore, by subtracting 6 from the right side of our equation, we have
Original equation: y = 2x^2 + 3x - 4 and our New equation: y = 2x^2 +3x -10
Let us investigate is this is the correct path to our solution.

Seems like we made it worse (because we did!). Let us evaluate our thinking about the problem of moving the parabola's vertex into the second quadrant. If we subtract 6 from y, that is equivalent to adding 6 to the right side of the equation. Therefore, the y-value at a certain x- value will be increased by an upward shift of 6.
Original Equation: y = 2x^2 +3x -4 New equation: y = 2x^2 + 3x + 2
Using Graphing Calculator, let us investigateif the new equation moves the vertex of the parabola into the second quadrant.
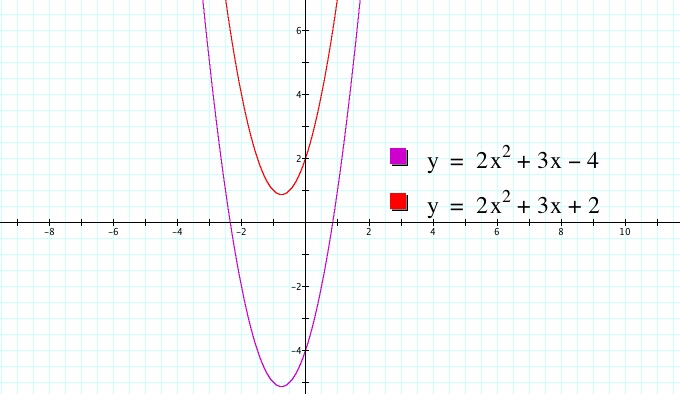
The new equation's vertex is now in the second quadrant!
Now, we should try to change the equation to produce a graph concave down that shares the same vertex.
We know that the graph is concave upward because the coefficient of the quadratic term is greater than zero, and in order to make the parabola concave downward we need the coefficient of the quadratic term to be negative. The question is - Will those two equations share the same vertex? Let's investigate with Graphing Calculator!
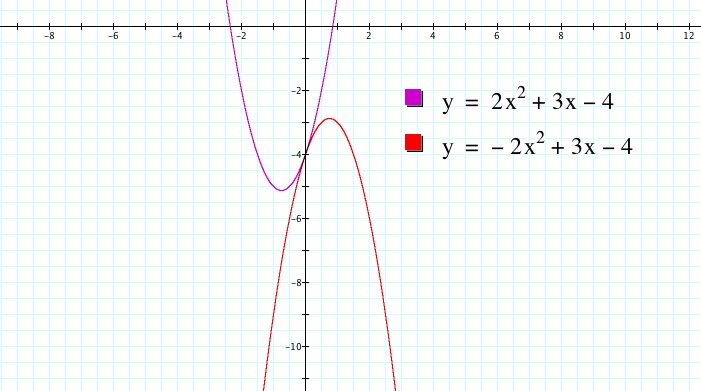
By making the coefficient of the quadratic term negative, the resulting graph was concave downward. Unfortunately, the two equations do not share the same vertex. If we take the original equation and write it in standard form, then it might become more apparent how we need to change the equation in order to produce the desired effect of finding an equation of a parabola that is concave downward and shares the vertex of our original equation.
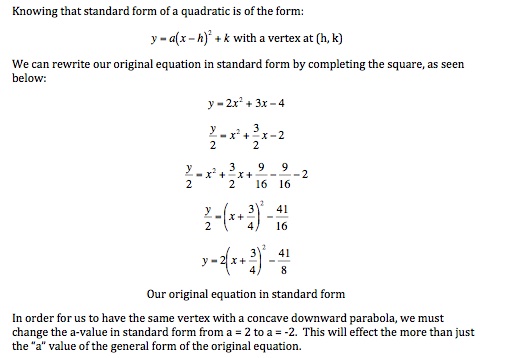
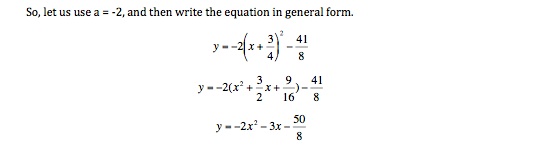
Now, let us try graphing this new equation and compare it to our original equation.
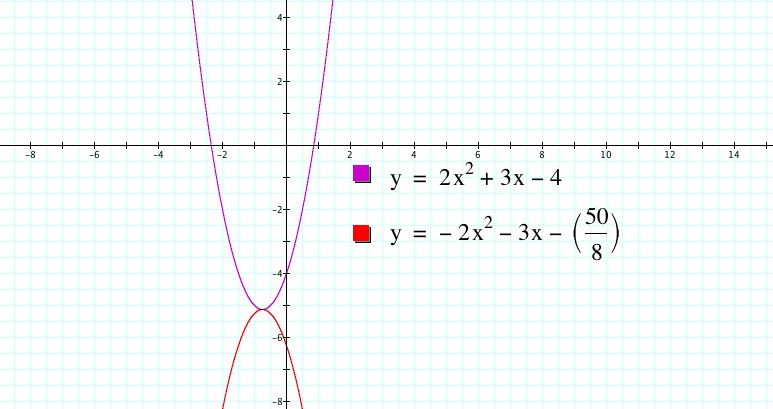
It worked! Why? Let's try to generalize. When we needed to move the concave down parabola, we needed to shift the function to the left by 1.5 units and down by 2.25 units. While we were able to accomplish this with completing the square, we could have easily realized from our previous work that we simply needed to add 1.5 units to each x and 2.25 to each y. By doing that, our result would be the exact same equation we found by completing the square. Therefore, it is interesting to develop an understanding of the benefits of using standard form and/or general form and the rich mathematical connections between the two.
Return Home.










